Probability and Statistics Lecture 9

Continued

Probability and Statistics Lecture 8

Expectation of a Random Variable

Expectation of Random Variable $X$.

Expectation, also called expected value, is a fundamental concept in probability and statistics. It provides the average or mean value of a random variable if the experiment were repeated infinitely many time.

For Discrete Random Variable

$$\text{X is a discrete r.v, },E(X)=\sum x(PX=x)$$

For Continuous Random Variable

$$\text{X is a continuous r.v, },E(X)={\int }_{-\infty }^{\infty }x(PX=x)$$

Properties

• $E(aX+b)=aE(X)+b$ Where $a,b$ are constant.
• $E(a)=a$
• Let $Y=g(x)$
  • $E(Y)=E(g(x))=\sum g(x)\times P(x)$ $=\int g(x)\times P(x)$
    • $E(X^2)=\sum x^2\times P(x)$

---

Variance of Random Variable $X$.

Formula for Variance

$$V(X)=E(X^2)-[E(X){]}^2$$

When $X$ is Discrete

$$V(X)=\sum [X^2\times P(X)]-[\sum X\times P(X){]}^2$$

When $X$ is Continuous

$$V(X)={\int }_{-\infty }^{\infty }{x}^{2}f(x)dx-{\left[{\int }_{-\infty }^{\infty }xf(x)dx\right]}^2$$

Alternate Formula for Variance

$$V(X)=E[(X-E(X){)}^2]$$

Properties of Variance

1. $V(a)=0$ where $a$ is a constant.
2. $V(aX)=a^2\cdot V(X)$ where $a$ is a scalar.

---

Standard Deviation for Random Variable $X$.

Variance measures the spread or dispersion of a random variable’s values around its mean. It quantifies how much the values of a dataset or distribution deviate from the expected value (mean).

Formula for Standard Deviation

The standard deviation (${\sigma }_X$) is the square root of the variance:

$${\sigma }_X=\sqrt{V(X)}$$

When $X$ is Discrete

$${\sigma }_X=\sqrt{\sum [X^2\times P(X)]-{\left[\sum X\times P(X)\right]}^2}$$

When $X$ is Continuous

$${\sigma }_X=\sqrt{{\int }_{-\infty }^{\infty }{x}^{2}f(x)dx-{\left[{\int }_{-\infty }^{\infty }xf(x)dx\right]}^2}$$

Alternate Formula for Standard Deviation

$${\sigma }_X=\sqrt{E[(X-E(X){)}^2]}$$

---

Standard $uv$ Rule Application in $E(X^2)$

Expectation Formula for Continuous Random Variable

The expectation of $X^2$ is given by:

$$E(X^2)=\int x^{2}f(x)dx$$

Applying the Standard $uv$ Rule

Using integration by parts ($uv$ rule), where $u=x^2$ and $v^{\prime }=f(x)$:

1. Let $u=x^2$, so $u^{\prime }=2x$.
2. Let $v^{\prime }=f(x)$, so $v=\int f(x)dx$.

Applying the integration by parts formula:

$$\int x^{2}f(x)dx=x^2\int f(x)dx-\int \left[2x\cdot \int f(x)dx\right]dx$$

Polynomial Behavior

Since $x^2$ is a polynomial, and differentiation reduces the degree of a polynomial, repeated application of the $uv$ rule will always result in a polynomial that eventually terminates. Therefore, the integration will conclude in a finite number of steps.

References

• Lectures at MPSTME
• Solve problems at Probability and Statistics Lecture 9

Information

• date: 2025.03.13
• time: 08:15

Link to original

Questions

These questions are based on the expectation of a random variable and PMF.

---

Example 1

Find $PMF$ of $Y=X^2+1$ and $E(X)$

Solution

Given Data:

X	-1	0	1
P(X=x)	$\frac{1}{2}$	$\frac{1}{4}$	$\frac{1}{4}$

The function given:

$Y=X^2+1$ Values of Y:

• When $X=-1$, $Y=(-1{)}^2+1=2$
• When $X=0$, $Y=(0{)}^2+1=1$
• When $X=1$, $Y=(1{)}^2+1=2$

Probability Calculation: $P(Y=1)=P(X=0)=\frac{1}{4}$ $P(Y=2)=P(X=-1)+P(X=1)=\frac{1}{2}+\frac{1}{4}=\frac{3}{4}$ Summary Table:

Y	1	2
P(Y=y)	$\frac{1}{4}$	$\frac{3}{4}$
Solving for expectation

$$E(x)=\sum xp(x)$$ $$=-1\times 1/2+0\times 1/4+1\times 1/4$$ $$=3/4$$ $$E(X^2)=\sum x^2\times P(x)$$ $$E(X)=3/4$$

---

Question 2

Given Probability Density Function (PDF):

$$f(x)=\{\begin{array}{ll}\frac{1}{4}{e}^{-x/4},& x>0\\ 0,& \text{otherwise}\end{array}$$

Problem Statement:

Find the mean and variance of $X$.

Solution:

The expected value (mean) of $X$ is calculated as:

$$E(X)={\int }_0^{\infty }xf(x)dx$$

Substituting the given PDF:

$$E(X)={\int }_0^{\infty }x\cdot \frac{1}{4}{e}^{-x/4}dx$$

Factor out the constant $\frac{1}{4}$:

$E(X)=\frac{1}{4}{\int }_0^{\infty }x{e}^{-x/4}dx$

Use integration by parts with $u=x$ and $dv=e^{-x/4}dx$:

$du=dx$ $v=-4e^{-x/4}$

Apply the integration by parts formula:

$\int udv=uv-\int vdu$

$E(X)=\frac{1}{4}\left({-4x{e}^{-x/4}|}_0^{\infty }+{\int }_0^{\infty }4e^{-x/4}dx\right)$

Evaluate the boundary term:

${-4x{e}^{-x/4}|}_0^{\infty }=0$

Simplify the remaining integral:

$E(X)=\frac{1}{4}{\int }_0^{\infty }4e^{-x/4}dx$

$E(X)={\int }_0^{\infty }{e}^{-x/4}dx$

Evaluate the integral:

${\int }_0^{\infty }{e}^{-x/4}dx=4$

Final calculation:

$E(X)=4$

Thus, the expected value $E(X)$ is $4$.

Steps to Solve:

1. Use integration by parts to solve the integral for $E(X)$.
2. Compute the variance:

$$\text{Var}(X)=E(X^2)-[E(X){]}^2$$

Question 3

Solve q10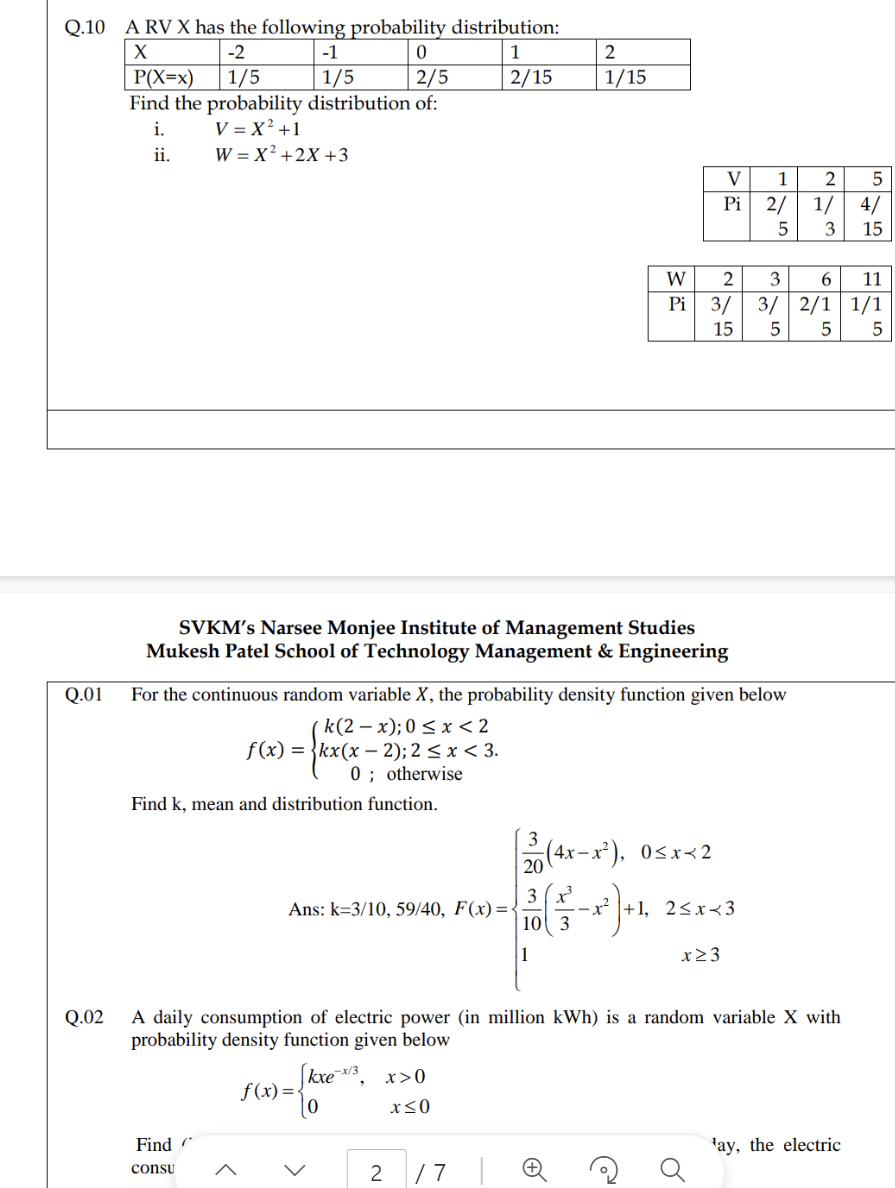

References

Information

• date: 2025.01.24
• time: 11:08

Continued

Probability and Statistics Lecture 10